How to Find the Integral of a Fraction
Click the blue arrow to submit. Notice that right now the right side is factored by coefficients.

Function Integration By Partial Fractions Overview Examples Video Lesson Transcript Study Com
Advanced Math Solutions Integral Calculator advanced trigonometric functions.
. The Integral Calculator lets you calculate integrals and antiderivatives of functions online for free. 1 x 2 3x 2 dx 1x2x1 dx. Some preliminary results 2 3.
Sometimes we can work out an integral because we know a matching derivative. You can substitute this variable into the expression that you want to integrate as follows. Now lets do another example.
And I am not quite sure how to integrate. Integration can be used to find areas volumes central points and many useful things. From this example we see that the arbitrary constant c is the fixed.
U d v u v-. We have already seen that any cost function for this marginal cost must be of the form C x x 2 a for some constant a. 2 1 2w5 w.
Algebraic fractions with a repeated linear factor 6 5. Indefinite integrals are functions while definite integrals are numbers. Instead of factoring by the coefficients.
Remember that the derivative of 2x-3 is 2 thus to take the integral of 1 2x-3 we must include a factor of 12 outside the integral so that the inside becomes 2 2x-3 which has an antiderivative of ln 2x3. Ex dx ex C 10. The Integral Calculator solves an indefinite integral of a function.
Sin x dx -cos x C 4. There are two types of fraction integrals you might come across. Integration by parts formula.
Sec x tan x dx sec x C 8. In the previous post we covered substitution but substitution is not always straightforward for. In the case in which degree P x degree F x it is necessary to do the polynomials division to obtain.
Algebraic fractions with two linear factors 3 4. Xn dx xn1 n1 C. Cos x dx sin x C 7.
Ax dx axln. 1 3 6x25x 2dx 3 1 6 x 2 5 x 2 d x. Lets start off with an integral that we should already be able to do.
Notice that the expression cos x dx still remains and needs to be expressed in terms of u. Given the example follow these steps. 1x2 and 5x3 are called the partial fractions of 6x13x25x6.
In this section we are going to look at quite a few integrals involving trig functions and some of the techniques we can use to help us evaluate them. Thus the cost function is given by C x x 2 500. Type 1 Factorisable denominator.
You must split it into two fractions using the method of partial fractions. Finally note the difference between indefinite and definite integrals. Declare a variable as follows and substitute it into the integral.
The following are the commonly used formulas used to solve problems related to integration. 1 dx x C 3. 3 x 4 A x 2 B x 3 displaystyle 3x-4A x2B x-3 2.
Example 2 Evaluate each of the following. Type in any integral to get the solution steps and graph. Cos x cot x dx sec x C 9.
Free integral calculator - solve indefinite definite and multiple integrals with all the steps. Show activity on this post. A dx ax C 2.
By using this website you agree to our Cookie Policy. Free Partial Fractions Integration Calculator - integrate functions using the partial fractions method step by step This website uses cookies to ensure you get the best experience. Example Here we write the integrand as a polynomial plus a rational function 7 x2 whose denom-.
Again this is because the derivative of. C 0 500 0 2 a a we have a 500. Cosxsin5xdx u5du using the substitution u sinx 1 6 sin6xc cos.
Recall that the complex number so that and. In addition if two complex numbers are equal then their real and complex components are equal. Fractions where the denominator is factorisable and fractions where it is not.
It is often used to find the area underneath the graph of a function and the x-axis. Lets work some more examples. It makes certain integrals much easier to do and.
First reduce1 the integrand to the form Sx Rx Qx where R Q. The first rule to know is that integrals and derivatives are opposites. You can also get a better visual and understanding of the function and area under the curve using our graphing tool.
74 Integration by Partial Fractions The method of partial fractions is used to integrate rational functions. Partial fraction decomposition for rational functions trigonometric substitution for integrands involving the square roots of a quadratic. Begin by factoring the denominator getting.
It is used in the Laplace transform which we meet. B Hence find 4 3 x x 2 x 2 1 d x. Find a partial fractions decomposition for.
When the integrand matches a known form it applies fixed rules to solve the integral e. Dealing with improper fractions 7. Choose Evaluate the Integral from the topic selector and click to.
Multiply both sides by the denominator of the original fraction in order to get rid of all denominators. X 4 x 2 5 x 3 d x. And try to find the fractions whose sum gives this result then the two fractions obtained ie.
A Show that 4 3 x x 2 x 2 1 can be written in the form A x 2 1 B x x 2 1 and find the constants A and B. Differentiate the function u sin x. We decompose fractions into partial fractions like this because.
So first try and factorise the denominator. Let u sin x. N -1 5.
That is we want to compute Z Px Qx dx where P Q are polynomials. I tried to split them into. Integrate algebraic fractions by first expressing them in partial fractions integrate algebraic fractions by using a variety of other techniques Contents 1.
For a I found that B 2 and A 2. 0 4 tt2 dt 4 0 t t 2 d t. Sec2 x dx tan x C 6.
Find the corresponding cost function C x. In a more general way the integrals of the kind R x d x P x F x where P x and F x are polynomials.

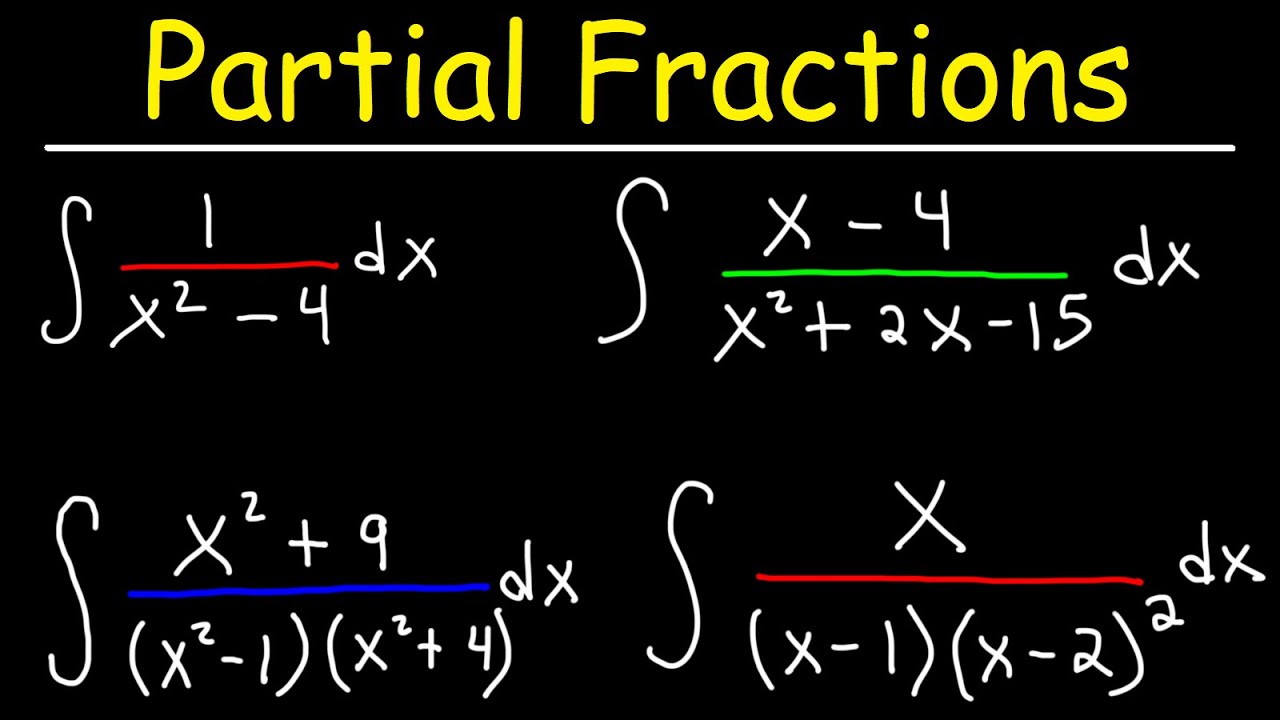
Example Integrating A Fraction With A Quadratic In The Bottom And A Linear Function In The Top Youtube

Integration By Partial Fractions Youtube
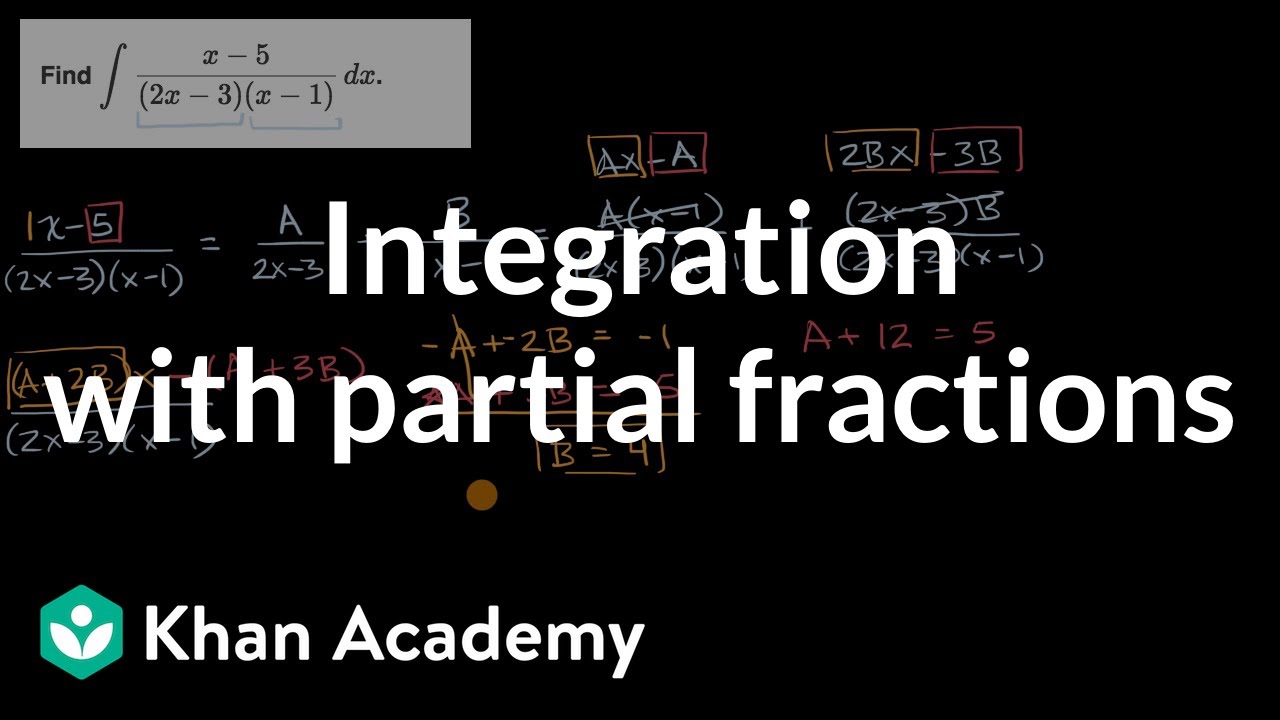
Integration With Partial Fractions Video Khan Academy

Integration By Partial Fractions Different Forms Videos Solved Examples
No comments for "How to Find the Integral of a Fraction"
Post a Comment